An Introduction To Differential Geometry (Part 3)

 Curves in 3-Space:

As previously defined, every point in the space R ₃ is uniquely determined by a position vector x = (x₁ ,  x₂ , x₃).  Then in order to introduce the concept of a curve we use a real vector function: x =  x(t)  and this will have components:

x₁ =  x₁ (t)             x₂ =  x₂ (t)                x₃ =  x₃ (t)

Which are single-valued functions of  real variable t defined in the interval:   I:   a  <  t  <   b.  To every value of t a point of R ₃  is associated whose position vector is x(t).

Then the vector function determines a point set M in R ₃   which we call a parametric representation of the set M, and the variable t is called the parameter of the representation. Given the above and that the trivial case of M = 1 point is discarded while the vector function must have multiple derivatives, we may assume:

1)  The functions x_i =  x_i (t) (i=1,2,3.....)  are  r (> 1) times continuously differentiable in I where the value of r will depend on the problem under consideration.

2) For every value of t in I, at least one of the three functions:

x_i (t) =   d x_i (t)/ dt

is different from zero.

Definition: Arc of a curve:

A point set in space  R ₃  which can be represented by the allowable representations of an equivalence class is called an arc of a curve.  The functional correspondence of the points of an arc to the value of t- given by an allowable representation x = x(t) is continuous.   

 If an arc is simple the correspondence between the points and the values of the parameter t is one to one.  In this case not only is the functional relation of the points to the values of t continuous but also the inverse relation, i.e. the relation of the values of t to the points.

Definition:  A curve:

A point set is called a curve if it can be represented by an equivalence class of the form x = x(t) whose interval I is not assumed to be closed or bounded, but is such that one always obtains the arc of a curve if the values of the parameter t are restricted to any closed and bounded subinterval of I.  

A curve is said to be closed if it possesses at least one representation which is periodic, i.e.. of the form:  x(t  +  w  ) =  x(t)

Thus the circle:  x(t) =  (r cos t, r sin t, 0)  with  r ²  =  4  is an  example of a simple closed curve.  

Thus, we have: x1 = r cos t ,  x ₂ = r sin t,  x ₃  = 0

->  x ₁ ²    +    x ₂ ²  =   r ² (cos ² t   +   sin ² t) =  4

So, the circle is closed and in the x ₁ x ₂ -plane, e.g.

Special curves:  A few special curves and their representations are now examined.  The main proviso here is to point out that there are curves of the form  x =  x(t)  that cannot always be represented as a whole in the forms:   x ₂  =  x ₂  (x ₁),   x ₃  =  x ₃  (x ₁).  This difficulty will occur when one value of the independent variable corresponds to several values of the dependent one, since the concept of a function requires a 1:1 onto correspondence of dependent to independent variable. Thus, in the case of the circle, a representation of the form just highlighted would be:

x ₂  = + Ö ( r ²   -   x ₁ ²²)      x ₃  = 0

If we choose the + sign, we have a semicircle in the upper half plane. If we choose the negative sign we have a semicircle in the other half plane.

Ellipse with center at origin of coordinate system in space.  In the parametric representation we can write (analogous to that for the circle):  x(t) =  (a cos t, b sin t, 0)  

Or:

x ₁ ² / a ² +    x ₂ ²  / b ² = 1,   x ₃  = 0

If the principal axes have lengths 2a and 2b (say with a = 8, b = 16), respectively and coincide with the x ₁  and  x ₂  axes, respectively,  we obtain the graph shown below:

And note that if a= b = r we just recover a circle:

 x(t) =  (r cos t, r sin t, 0)

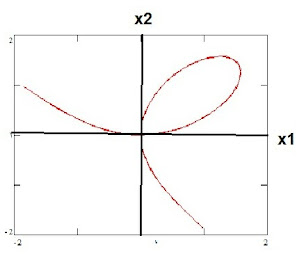
The folium of Descartes:  This can be expressed in parametric form as:  

 x(t) =  (3t/ 1 +  t³ ,  3t²/ 1 +  t³  , 0)  

This curve will be found to lie in the 1st, 2nd and 4th quadrant  of the   x ₁ -  x ₂ plane as shown below:

By inspection the reader will also see it has a double point at (x ₁ ,  x ₂) = (0, 0) .  Note also that part of the curve in the 2nd quadrant corresponds to values of t from -1 to 0, while the loop in the first quadrant corresponds to values between 0 and  ¥.  In the 4th quadrant the t-values range from - ¥  to  -1.

The circular helix:  

The parametric form here is:  x(t) =  (r cos t, r sin t, ct)   c ≠ 0.  The orthogonal projection of the helix into the   x ₁ x ₂ - plane, e.g.

 is the circle: 

x ₁ ²  + x ₂ ²     -  r ²  = 0,  x ₃  = 0

which is the intersection of this plane with the cylinder of revolution on which the helix lies. Projecting the helix orthogonally into the x₂ x ₃ - plane we obtain the sine curve:

 x₂ - r sin (x ₃  /c)  = 0,  x ₂ = 0

A cosine curve will be obtained by projecting the helix orthogonally into the x ₁ x ₂  - plane.

Suggested Problems:

1) Sketch the graphs of;

x ₂  = - Ö ( 8 ²   -   x ₁ ²²)

And:

x ₂  = - Ö ( 16 ²   -   x ₁ ²²)

On the same Cartesian axes

2)(a) Write the polar form of the equation of the line:

3 x ₁   +   4 x ₂   =  5

b)Determine the polar (r,  q) equation for :

x ₁ ²  + x ₂ ²   -  2ax ₂  =  0,    a  ≠   0

And sketch the resulting curve

3)(a)  Let r and  q  be polar coordinates in the x ₁ x ₂ - plane. Give the representation of the following curve in Cartesian coordinates:

r =   a q

And sketch it.

b)  A student's analysis of the curve (cardioid):

 r =   6 (1 - cos q),    is shown below:

Using differential calculus show how an expression for the angle y  can be obtained in terms of the angle   q.