In 1976 three scientists (James Hays, John Imbrie, Nicholas Shackleton) published a seminal paper. In it they presented a deep sea "climate record" which they claimed contained the same temporal cycles as three parameters shown in Fig. 1 below, from a recent paper appearing in Physics Today (May, p. 48) by Mark Maslin.
Their technique basically entailed using deep sea sediment cores to extract marine micro-fossils, to then estimate past sea surface temperatures. The claim then was made that this geological proxy climate record revealed the same temporal cycles manifested in the three orbital parameters: eccentricity, obliquity, and precession. All this is to substantiate the so-called Milankovitch theory which asserts these three distinct cycles are applicable to Earth's orbital motion.
The eccentricity (a) describes the shape of Earth's orbit around the Sun, said to vary from near circular (as it is now) to more elliptical, with a period of 96,000 years. The obliquity (b) is the tilt of the Earth's axis of rotation with respect to the orbital plane, and oscillates with a period of 41,000 years. The precession (c) combines the spin of Earth's orbital axis and its orbital path over time, producing a 21,000 year cycle.
Let me now back up to say I do not regard this as a "theory" but rather a hypothesis. A theory, as I (and most physicists) define it, refers to a hypothesis which actually has made predictions that have been verified. To my knowledge no such predictions have been made by Milankovitch's acolytes. To be consistent, one would also expect such predictions to come out of a pure model from celestial mechanics - not one dependent on sea floor topography or geology. These are two distinct sciences after all. (Paleo-climatology, i.e. based on marine mciro-fossils, is also distinct from celestial mechanics.)
Start with its contention that the obliquity of the ecliptic (inclination of Earth to its orbital axis) varies from 21 to 24 degrees over a 41,000 period in a process called ‘nutation’. This is certainly a magnitude in excess of a half degree (1800”) on either side of its current 23.5 deg.
Astronomers-astrometrists recognize no such period or differential of axial tilt. The following is from the book, Astronomy- Principles and Practice by A.E. Roy and D. Clarke, 1978, Adam Hilger Books, p. 118:
“Because of the nutational wobble in the Earth’s axis of rotation, the obliquity of the ecliptic (KP in Fig. 10.32) varies about its mean value. The magnitude on either side is about 9.”2.”
For the benefit of non-astronomers, the magnitude cited (9.”2) isn’t even one hundredth of a degree! Indeed it is nearly a factor 4 LESS than a hundredth of a degree! (which translates to 36”- there are 3600” = 1 degree))
Going now to Eichhorn and Mueller’s standard text in astrometry and geodesy,
p. 69, “astronomic nutation’:
"The main term of astronomic nutation is produced by the non-coincidence of the Moon's orbit with the ecliptic in conjunction with the retrograde motion of the lunar nodes. This results in a periodic change in the obliquity of the ecliptic termed nutation in obliquity, denoted by d e
The astronomic nutation, from now on called simply ‘nutation’ is not to be confused with the true nutation appearing as a force-free precession (Eulerian motion) of the Earth’s rotation axis about its principal moment of inertia axis, which is part of the polar motion
The first six terms of the expression for nutation in obliquity are:
(9.”2100 + 0.”00091t) cos W - (0.”0904 - 0.”0004t) cos 2W – (0.”0024 cos (2 w m + W) + 0.”0002 cos (2 w s – W) + 0.”0002cos 2 ( w m + W) + (0.”5522 – 0.”00029) cos 2W s
Where t denotes the time interval measured from 1900 January 0.5 d ET in Julian centuries (1 JC = 36525 mean solar days), W is the longitude of the mean ascending node of the lunar orbit on the ecliptic measured from the mean equinox of date, w m is the ‘argument’ of the point where the Moon is nearest the Earth (i.e. from the lunar perigee), w s is the mean longitude of the solar perigee measured from the mean equinox of date, and W s is the geometric mean longitude of the Sun measured from the mean equinox of date.
We can then examine the putative change in the eccentricity e, which is currently at 0.0167 but which Milankovitch adherents claim can reach e = 0.07. (Elongating the orbit so as to influence climatic factors, e.g. see this video demonstration:
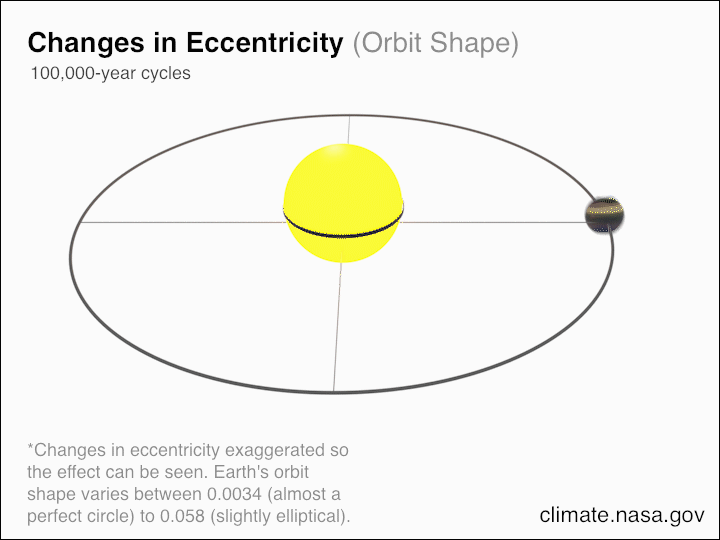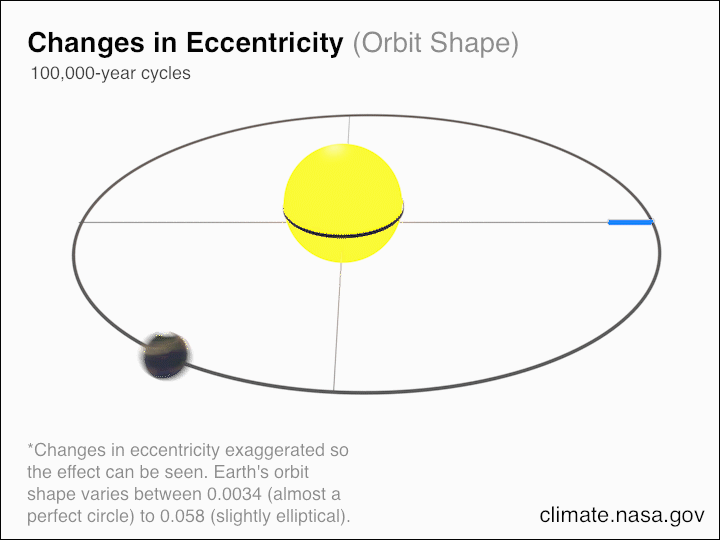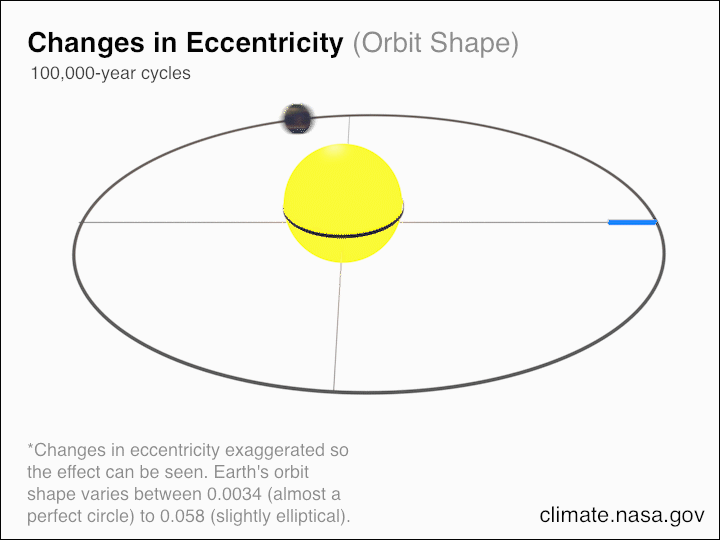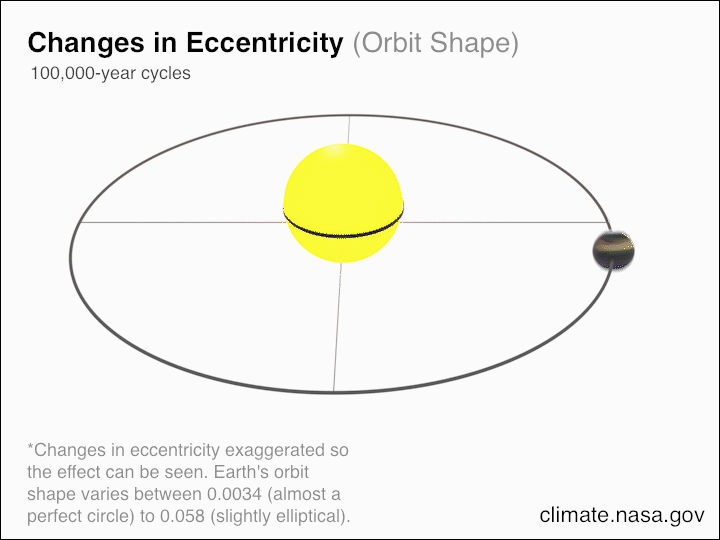
But what would it take to change the shape of the Earth's current orbit, to the one proposed by Milankovitch supporters? How much energy would be required? We can use the equation for the total energy of the orbit, in terms of the eccentricity, e, and the dimension of orbit known as the semilatus rectum, r o (= b
2 /a) and the other familiar parameters for Newtonian orbit computations, e.g.
The objective is to find the change in energy E, i.e. when e is altered from 0.0167 to 0.07. This turns out to be approximately:
D E » 1.9 x 10 42 J
This is an enormous amount of energy input. WHERE does it come from? HOW is it produced? What is the time evolution - in energy increments- leading to the final more eccentric orbit? The Milankovitch adherents who adopt the work of a Serbian civil engineer, provide no answers. How can they when they are working from reconstructed sea floor geology findings and not celestial mechanics per se?
Given the preceding we should acknowledge that there are significant problems with that Serbian civil engineer's hypothesis that bid us to exercise much more caution, i.e. before adopting it
without qualification. Especially given its reversed engineered construction from geological data, to arrive at a celestial mechanics format requiring new orbital elements. Or conferring the benediction of an origin in celestial mechanics, as opposed to geology. One major issue was raised in a paper by Daniel B. Karner and Richard A. Muller 2 which pointed out a number of serious problems, inconsistencies
associated with the Milankovitch hypothesis.
Specifically the authors –
after assessing the data from numerous sources (e.g. U-Th dating
from the Devil’s Hole cave in Nevada) found that:
-
the Devil’s Hole data indicated a shift in d 18 O to interglacial values and was “essentially complete by 135 ka but the
Northern Hemisphere summer insolation hadn’t yet warmed to the point when it
would have triggered anything extraordinary, let alone a glacial termination” Adding: “We
call this discrepancy the causality
problem”
-
Most
of the sea level rise took place prior to the expected insolation warming
The
authors conclude (op. cit.):
“The standard Milankovitch insolation theory
does not account for the termination of the Ice Ages.”
Adding
that:
“We can conclude that models that attribute
the terminations to large insolation peaks (or equivalently to peaks in the
precession parameter)…are incompatible with the observations.”
Equally
significant, perhaps, Richard A. Muller
has pointed out in a separate paper
3 that “the spectral shapes predicted by the
Milankovitch theory do not match those in the spectrum and bispectrum of the
data..”
In
addition, Muller notes that the net forcing associated with clouds, i.e. about
30 W/m 2 is substantially greater than the rms
variations in insolation. This leads
him to conclude: “The changes in cloud
cover could be more important than the changes in Milankovitch parameters.”
Finally,
Puetz et al4, employed a “universal cycle model” – using a Universal
Wave Series (UWS) with cycles in the kyr range - to show that
orbital tuning limits independent and objective testing of an empirical
hypothesis like that of Milankovitch. In effect, while Milankovitch offers a “seductively elegant solution to the problem
of age-stratum mapping”, its template remains a trap for selection effects
(and data bias) to enter. This results in a condition, as it pertains to the
Milankovitch hypothesis, whereby:
”Reporting
bias occurs when articles mention favorable results from orbitally tuned
records, which are supportive of the Milankovitch theory, while failing to
mention unfavorable results from un-tuned versions of the same records.”
The
reason is clear given that (ibid.): “Statistically
significant positive results that support a desired outcome are more likely to
be published in high impact journals.”
Thus,
while one can certainly appreciate Prof. Maslin’s article, it is also
worthwhile to be aware of the problems to do with the underlying hypothesis, most of which I suspect were engendered by the origins in sea floor geologic data..
My primary gripe, then, is there is no independent confirmation of the Milankovitch hypothesis from celestial mechanics proper. That is, where is the model, or even numerical simulation, actually showing how the three orbital parameters evolve over time t to yield the temporal cycles suggested by the marine microfossil studies? Granted Hays et al's paper is ingenious - even somewhat convincing - but when you strip away all the ersatz arguments it is still reverse engineered geology, from which the changing orbital elements must be indirectly inferred.
Where is the direct connection to orbital parameter development in a celestial mechanics context? In particular, leave out the geological data, where is the theory based on celestial mechanics showing how Earth's orbit changes its parameters to yield the temporal cycles claimed by Maslin, as well as Hays et al, and oh yes, Milankovitch.
References:
1.
J.D. Hays, J. Imbrie, N.J. Shackleton, Science
194, 1121 (1976)
2.Daniel
B. Karner, Richard A. Muller, A Causality
Problem for Milankovitch, Science,
288, 2143 (2000)
3.
Richard A. Muller, Limitations and
Failures of the Milankovitch Theory, poster paper, American Geophysical
Union Fall Meeting (2001)
4.
Stephen J. Puetz, Andreas Prokoph, Glenn Borchardt, Evaluating Alternatives To The Milankovitch Theory, Journal of
Statistical Planning and Inference, 170,
158 (2016)
